MATHEMATICAL ANALYSIS

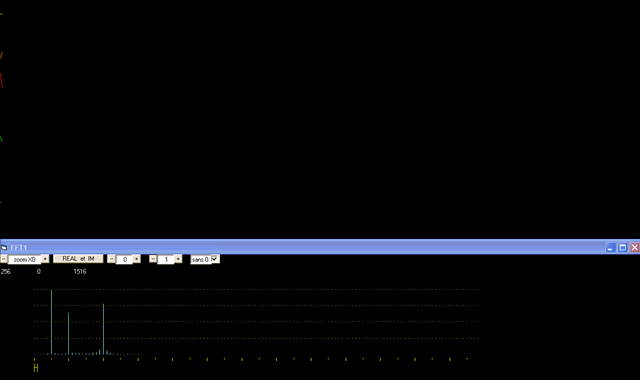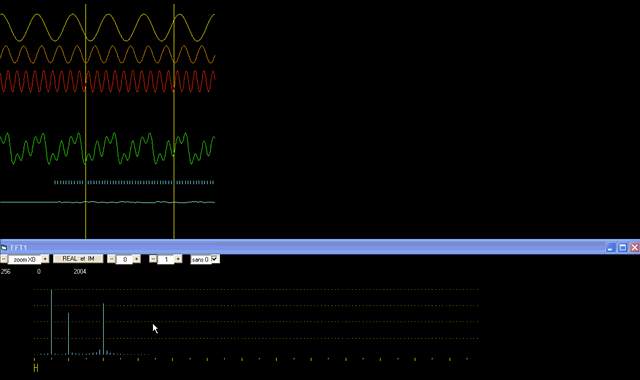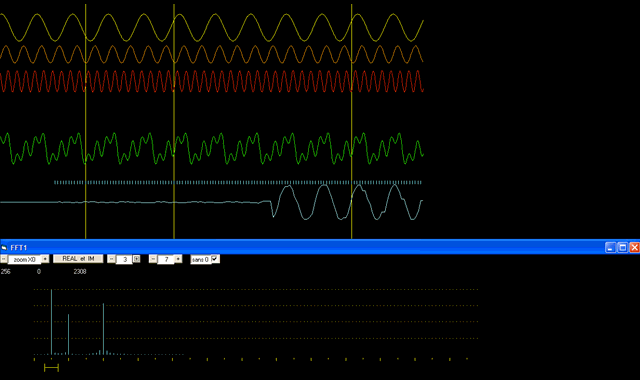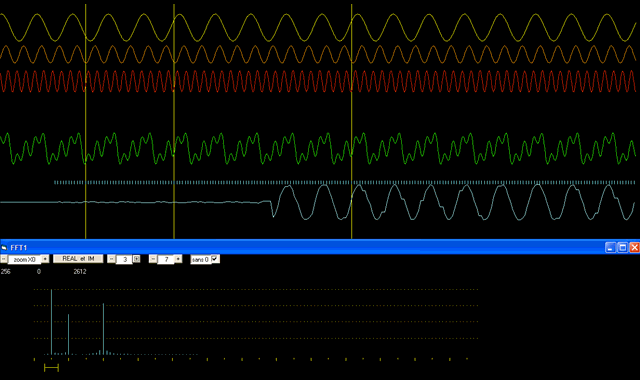
The FFT ou Fast Fourier Transform is a mathematical algorithm allowing to extract the different frequencies componants from a wave.

The green curve has 3 differents componants:

• the yellow curve : 5 cycles/time unit
• the orange curve: 10 cycles/time unit
• the red curve: 20 cycles/time unit

By applying a Fourier transform to the green curve, we obtain three peaks corresponding respectively to frequencies 5, 10 and 20 (in the graph below).

The position of the peaks gives the frequency of the wave. The size of the peak gives the size (amplitude) of the wave.

Starting again from these peaks, another mathematical process (the inverse Fourier transform) allows us to recreate the wave corresponding to the desired peak : the blue curve recreates the curve corresponding to the first peak of 5 cycles /time unit. The blue curve, although extracted from the green curve recreates only its first component (the yellow one).